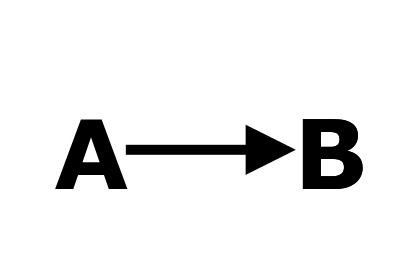Reading causal loop diagrams can be a little counter-intuitive. It will be important to understand how to read them in order to understanding the dynamic quality of the models that will appear in subsequent posts. The interactions between the various elements and the effects of those interactions on stocks and flows are typically represented by a solid black arrow (Figure 1.)
"A" has an interaction with "B" and that interaction is in the direction of "A" to "B." But what's the effect of "A's" interaction with "B?" To display this effect, a green open head arrow or a red closed head arrow is used to describe the type of interaction between the two elements.
A green open-head arrow (Figure 2) is a direct relationship. A red closed-head arrow (Figure 3) is an inverse relationship.
To help understand these relationships, consider the analogy of being in the driver's seat of a car. Imagine the car has a constant speed of 40 miles per hour. The car has been designed to go this speed with your feet off the peddles. (Not a particularly safe design feature, I'll grant. But this is a thought experiment. So ride along with me for a little while. I promise we won't crash.) Now, when you increase (↑) pressure on the gas peddle the car's speed increases (↑). If you then decrease (↓) pressure on the gas peddle the car's speed decreases (↓) until it returns to the original 40 MPH. That's the direct relationship illustrated between "A" and "B" in Figure 2. As "A" increases, so does "B." Increase pressure on the gas peddle and the speed of the car increases. As "A" decreases, so does "B." Decrease pressure on the gas peddle and the car speed decreases until it slows down the the original 40 MPH. More of "A" results in more of "B" (↑↑) while less of "A" results in less of "B." (↓↓)
Now for the brake. If you increase (↑) pressure on the brake peddle the car's speed decreases (↓) – it slows down to something less than 40 miles per hour. Increase the pressure on the brake enough and the car will stop. However, if you decrease (↓) pressure on the brake the car's speed begins to increase (↑). If you remove all pressure on the brake peddle, the car returns to the constant 40 mile per hour speed. That's the inverse relationship illustrated between "A" and "B" in Figure 3. As "A" increases, "B" goes the opposite way and decreases. Increase pressure on the brake peddle and the speed of the car decreases. As "A" decreases, "B" goes the opposite way and increases. Decrease pressure on the brake and the speed of the car increases until it is once again moving at 40 MPH. More of "A" results in of less of "B" (↑↓) while less of "A" results in more of "B." (↓↑)
For an example of these relationships in action, let's look at the dynamics behind two cosmic quandaries: Which came first, the chicken or the egg and why do chickens cross the road?
No matter which came first, eggs come from chickens and chickens come from eggs. If the number of eggs increase, the number of chickens will increase. If the number of eggs decrease, the number of chickens will decrease (Figure 4.)
If the number of chickens increase, the number of eggs will increase. If the number of chickens decrease, the number of eggs will decrease (Figure 5.)
These are direct relationships as described for Figure 2. Taken together, the causal loop diagram is shown in Figure 6.
If times are good, there are more and more eggs leading to more and more chickens and Chicken World starts to get a bit crowded. In search of a better life, some of the chickens decide to cross the road (now you know why they cross the road!) Another direct relationship in the system (Figure 7.)
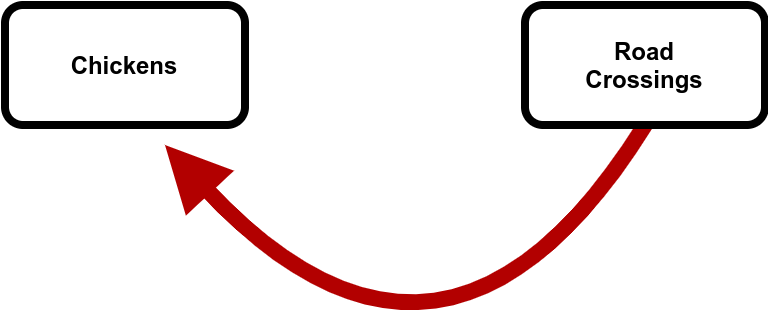
However, life is so good on the other side of the road that chickens never cross back to where they started. An increase in the number road crossings result in a decrease in the number of chickens. (Figure 8.) This is an inverse relationship as described for Figure 3.
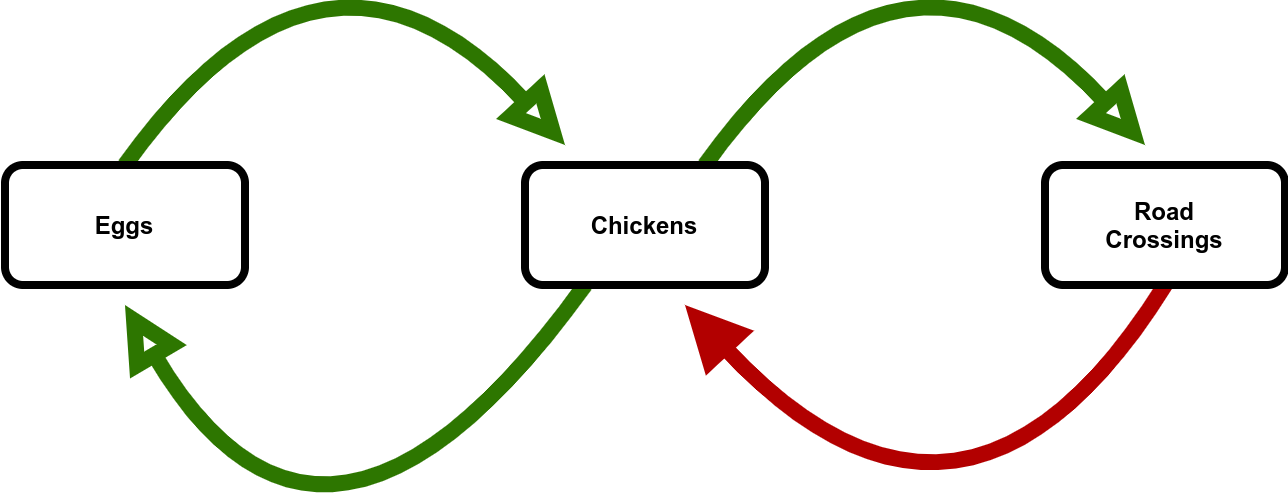
Connecting all the pieces, the very simple causal loop diagram describing Chicken World is shown in Figure 9.
This simple example illustrates how systems stay balanced. If there are too many eggs, leading to too many chickens, more chickens cross the road until the population is restored to a sustainable level. If too many chickens cross the road, the number of chickens decrease and therefore so do the number of eggs which means there are fewer chickens crossing road. Once again, the population is eventually restored to a sustainable level.
That's all the system dynamics you'll need to read the causal loop diagrams presented in subsequent posts.